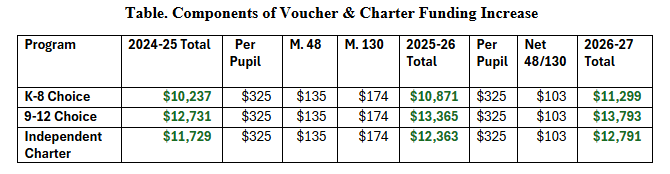
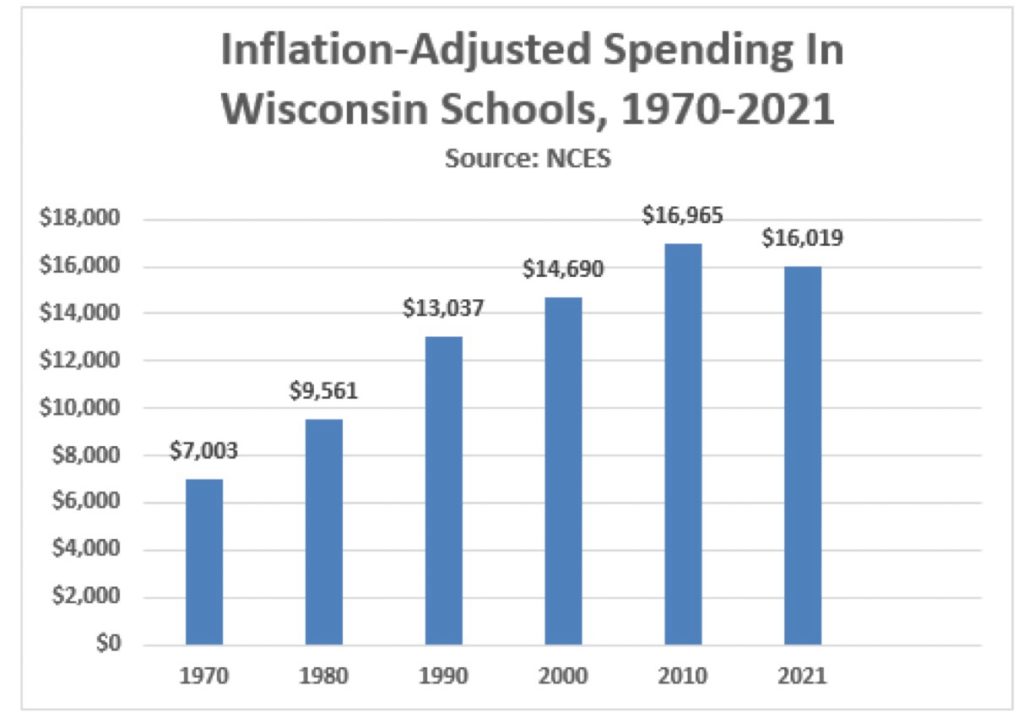
If you had told me at my Year 13 graduation, that I would be standing here today, I would have been extremely amused.
Let me start off by telling you a story.
18 years ago, at my own LHS graduation, the historian Robin Lane Fox was the guest speaker. If you don’t know Robin Lane Fox, his works on Early Christianity and Alexander the Great are fantastic.
Back in 2007, I was no esteemed guest, but rather, like most teenage girls, a jumble of competing interests and personalities. I had high hopes of becoming an archaeologist or historian myself, but due to my disobedient nature, including trying to sell this school in a local newspaper, I admit I barely made it through LHS.
But after luckily pulling it together for my A-Levels, when I got up on stage at graduation to shake Robin’s hand, I found that- with no notice or warning given to the audience and after shaking the hands of dozens of girls- Mr. Lane Fox kissed me on the cheek.
All eyes were on me as I walked back to my seat, as it was presumed, considering my naughtiness, that I had somehow done something to initiate the incident, or as my mother later put it it very much looked from the back of the hall that you had kissed him’
It was only towards the end of the ceremony that Lane-Fox mentioned that he had kissed the cheek of any girl who had graduated with A-Levels in the classics trifecta, Latin, Ancient Greek and ClassCiv, which had been my subjects.
When I was contacted to see if I would be the guest speaker here today, my decision rested on one condition. Was LHS still teaching classics and the arts with the same intensity? I looked at the curriculum online and saw that yes class’ civ and latin were still mandatory, with a few exceptions.
Having access to all STEM subjects and the full range of humanities, while also being a Steinway-accredited school of music, is such a gift.
To all the teachers and staff here, and to all those who have kept this school going for the last 175 years, I want to say thank you.
Thank you for keeping education glorious. There are many schools worldwide that have sacrificed the classics for the temporal educational trends of modernity, and you have remained resilient in providing thousands of girls with access to a timeless and historical education.
For every one of me here, there are hundreds of other girls whose lives you’ve enriched and who are endlessly grateful.
I could not appreciate the value of this education in my youth, just as you girls here can not fully grasp its significance today. Yet in later years, you may find that your encounters with literature or distant places carry a deeper resonance-words and concepts that once felt like burdens imposed by this very institution will reveal layers of meaning that others overlook.
The Latin inscriptions adorning a Roman church fresco’s ceiling, or the subtle tributes in novels to classical thinkers you once studied, will quietly illuminate a secret world.
The rewards of the education you have been given will not emerge in classrooms or exams, but in the unscripted encounters of a life well-lived.
I won’t kiss anyone today; instead, I will share the most important message I think I can give.
Make the most of this great education that you have been given.
I am just one voice of a thousand annoying experts and adults who will tell you how you should live your life. There will be plenty of warnings about what’s bad for you and how to protect your physical and mental health as you graduate into a complex and busy world.
Most people won’t risk telling you what’s good for you, but I’m up here perhaps only because l’ve taken more risks than my peers. I have been successful, and I also spent years living in a tiny office closet. I have been popular and I have felt hated. I have made millions, and I have lost millions. I’m proud of some of my work, yet I’ve spent many nights lying awake, cringing at ideas I once defended fiercely. Mistakes and failures litter my path, and each one taught a lesson that helped fine-tune my future actions.
And like all women, I have felt beautiful, and I have felt ugly.
It doesnt matter.
Nothing has mattered more in my life than cultivating beauty and philosophy. Spending time and energy seeking it out—in old buildings, paintings, poems, and old books, in the autobiographies of great humans, to find inspiration on how to live a good life.
Modernity moves quickly; what is timeless, by definition, has stood the test of time.
Traditional experts don’t want you to know that you can inject beauty into any moment as a conscious decision. Beauty isn’t defined by aesthetics; it’s about intention.
Make moments intentional. Make career decisions intentionally. Be very intentional with your time, yet when something feels off appreciate that you have all the agency to completely change it.
Take risks fully, intentionally. Spend time with people fully, intentionally, always with the notion that one day that person you’re spending time with may no longer be here. If you can, get to know your parents as if they are your friends, in them you may learn about yourself.
Travel and see the world, intentionally or recklessly. There are so many flavors of life, so many cultures, so many different types of living and people. For some of you, this part of England may be the best place for you to flourish, but make the decision to spend your life here consciously, with full awareness of how big the world is.
I am living testament that you don’t need family money to be able to travel or seek opportunities. During my gap year, [I] sold plastic garden sinks at trade shows to save up and travel to new lands.
In the end, I found my people and the culture where I belong on the other side of the world, in San Francisco, California. moved there originally at 24 years old with the ambition to launch an artificial intelligence start-up fund, which I did, kinda, though it was no big success.
I had no idea what I was doing.
But in the countless cold emails I sent and the essays I published I found not only friends, but people with similar visions who recognized mine, and over the last decade we have built projects and companies together.
San Francisco is a paradise of socially awkward tech lovers who throw awful parties while debating philosophy endlessly.
If that sounds like a place you’d fit in, reach out to me and I’ll show you around.
But your people might be scattered across places like London or Singapore, or they’re waiting for cities that don’t exist yet that need you to instigate and design them, or maybe they’re launching rockets toward Mars with dreams of new worlds.
You can’t know yet.
The world is an uncharted adventure waiting for you to explore.
Make the most of this freedom while also remembering that it is every generation’s responsibility to defend the same freedom for future generations. Over time, you might learn that such freedom is found in the opposite of whatever the media and news sell you.
Experts are nearly always wrong because expertise is a transient quality. Artificial intelligence is mostly wrong, too, because it is trained on the corpus of eternally fallible humans.
But if you shrug off the noise the world shoves at you, beauty and joy will slip in effortlessly. Elegance can be found in a semiconductor’s complexity, a violin concerto, a flower’s bloom, even a line of code. I’m not ashamed to admit that all of these things can move me to tears, and do often, daily.
If you really notice and take in the world, it’s overwhelming.
If you really notice and take in the world, it’s difficult to be depressed.
Back in 2007, I didn’t know what the future held for me, but I knew I wanted to try as many flavors of life as possible. And in 2025, I feel the same.
I could easily repeat the last ten years for the rest of my life, navigating technology companies and repeat opportunities that eventually become easy.
Or I could become a painter, or poet, a surgeon, a truck driver.
With the internet in your pocket, anyone can teach themselves a whole new field or career path at any time. Sometimes I spend weeks or months studying a niche area of research just for fun. I can tell you a lot about neurodegenerative diseases but I never learned to drive a car. Your choices don’t need to make sense to other people.
You are never too young or too old to start again, to move away, to restart your life, and to completely redesign your mind.
It’s the most freeing thought.
All your limitations, now and in the future, are self-imposed.
Thank you and .. good luck.